Rational Points on Elliptic Curves
Episode #8 of the course Great math problems for the 21st-century mind by John Robin
What if I asked you to find every rational point on our familiar curve, y2 = x3 − x? By this, I mean any number that is a fraction.
In fact, there are none. I will go further and claim there are none for:
y2 = x3 − 4x
y2 = x3 − 9x
y2 = x3 − 16x
But there are infinitely many for y2 = x3 − 25x. Check for yourself. To back my claim, I will give you (25/4, −75/8) for y2 = x3 − 25x as one of the points.
You can search all you want for a solution for the other three curves, and if you find a solution, then we must live in different universes with different laws of mathematics.
How can we know, though, exactly which elliptic curves have rational solutions and which have only irrational (not fraction) solutions?
In the example above, I gave you the associated curves for the congruent number problem: y2 = x3 − n2x, where n is a congruent number. This corresponds to the problem: What are all the triples of rational numbers whose squares form a common difference of n?
(In the case of the elliptic curve, y2 = x3 − 25x, which corresponds to n = 5, the answer is 31/12, 41/12, and 49/12—try squaring each of these numbers, subtracting the middle from the biggest and the smallest from the middle, and you’ll see the difference in each case is 5.)
The set of congruent numbers is itself an interesting problem. Through Tunnell’s Theorem, which itself relies on the Birch and Swinnerton-Dyer Conjecture being correct, we can determine the complete set of congruent numbers.
What this means is that we know if a number, n, is congruent, then all the elliptic curves of the form y2 = x3 − n2x have rational solutions (x,y).
Now, congruent numbers are just one example of triples that have associated elliptic curves. We are interested in knowing a criterion for all elliptic curves to determine if they have rational points or not. In order to do this, I will have to tell you a bit more about elliptic curves and modular forms.
You may recall from Lesson 3 that in order to prove Fermat’s Last Theorem, Andrew Wiles showed that the Frey Curve, y2 = x(x − an)(x + bn), did not have a modular form. What he actually showed was that the Frey Curve was non-singular.
To understand what I mean by non-singular, let me explain what we are looking for in an elliptic curve.
To help you picture this, here is our diagram of two non-singular (i.e., proper) elliptic curves:
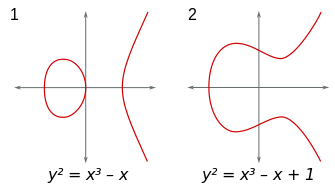
Each of these has three distinct roots. For curve 1, the roots (i.e., values for which y = 0) are: x = 0, x = −1, x = 1. For curve 2, the roots are a bit more complicated to find and involve using cubic root calculation methods, which you can investigate if you are curious, but suffice to say, two of these roots are complex numbers. You’ll notice in the picture, the graph only passes through the y-axis in one point and has two inward-bending cusps on the right, mirror images of each other. These warps in the graph correspond roughly to the two distinct complex roots.
How do we know if an elliptic curve has three distinct roots—i.e., is non-singular? We can check a formula called the discriminant. For any elliptic curve, y2 = x3 + ax + b:
Δ = −16(4a3 + 27b2)
is called the discriminant. When the discriminant is non-zero, there are three distinct roots. When the discriminant is zero, there are fewer, and the curve will not have a modular form.
For example, for the elliptic curve y2 = x3:
Δ = −16(4(0)3 + 27(0)2) = 0
We can tell at a glance that the root x = 0 is tripled—i.e., (x)(x)(x) = 0 has only the three possibilities of x = 0, x = 0, or x = 0. This is a singular elliptic curve, verified by the zero discriminant. It will not have a modular form.
In the case of Frey’s Curve and Fermat’s Last Theorem, Andrew Wiles, in showing that the curve was not modular, proved, in fact, that the discriminant was zero.
Understanding this, then, we can narrow down our search for rational points on elliptic curves to only those that are non-singular. To narrow them further, tomorrow, we will investigate some more about modular forms themselves on given non-singular elliptic curves, and this itself will lead us right up to the Birch and Swinnerton-Dyer Conjecture.
Recommended resource
Here is a link to the online encyclopedia for integer sequences that have all congruent numbers.
Recommended book
Rational Points on Elliptic Curves by Joseph Silverman
Share with friends

