From Sums to Curves to Contradictions: How Fermat’s Last Theorem Was Finally Solved
Episode #3 of the course Great math problems for the 21st-century mind by John Robin
How did Andrew Wiles, a Princeton University professor, progress from the basic claim—
There are no examples for any higher sum of powers, written in the form:
an + bn = cn
—to a 129-page proof that covers group theory, modularity theorem, and elliptic curves?
Actually, when Wiles began his graduate research in the summer of 1975, he was already entering the deep end of work created by mathematicians decades before him.
During the 1950s and 1960s, Japanese mathematicians Goro Shimura and Yutaka Taniyama laid the foundation by exploring the relationship between two things from the abovementioned cocktail of Wiles’s proof: modular forms and elliptic curves.
An elliptic curve is a curve that has the form:
y2 = x3 + ax + b
For instance, y2 = x3 − x and y2 = x3 − x + 1 are both elliptic curves, where a = −1, b = 0 for the first one and a = −1, b = 1 for the second one.
Here are their graphs to help you visualize them:
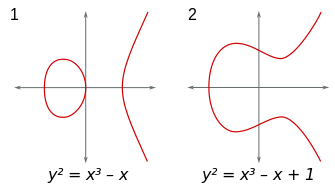
There are endlessly many elliptic curves, since you can make a unique one for every value of a and b you choose.
Now, where elliptic curves get really interesting is when you consider only rational values for the possible x,y pairs. Rational numbers are numbers formed by fractions like 1/3, 4/5, and 5 (5 is a fraction because it is 5/1).
If you limit yourself only to rational numbers for x,y, then you can ask the question: How many solutions (x,y) will you get for a given elliptic curve?
Take our example:
y2 = x3 − x
Let’s start by allowing ourselves all fractions formed by the numbers 1 and 2. That would be:
{0, 1, 1/2, 2, −1, −1/2, −2}
If we try all these numbers as possibilities for x,y in the elliptic curve equation, we will find (check for yourself) the following solutions:
(0,1), (0,−1)
Only two. Now, you can do this with all the fractions made with 1 through 3—that is: {0, 1/2, 1/3, 2, 2/3, 3, 3/2, 1, −1/2, −1/3, −2, −2/3, −3, −3/2, −1}. You can do this with 1 through 4 and so on. Every time, see if the increase adds more solutions. As you increase the number of fractions, with some curves, you will find further solutions to add to your collection.
This is related to a pattern that emerges as you increase your number range and analyze the number of solutions for a given (proper) elliptic curve.
There is an equation one can find for each elliptic curve that looks like this:
a1q + a2q2 + a3q3 + a4q4 + a5q5 + …
where a1, a2, a3, etc. correspond to the number of solutions you find for each number field of a given size (I will talk more about this in Lessons 7-9).
This equation is called a modular form. What Shimura and Taniyama explored then was whether or not a modular form is simply another form for an elliptic curve. In fact, they proposed that every (proper) elliptic curve must have a modular form.
Andrew Wiles was a specialist in modular forms. When he began his research, he was interested in a particular type of elliptic curve called a Frey Curve:
y2 = x(x − an)(x + bn)
Do you notice how the an and bn look a bit like the famous an + bn = cn? In fact, mathematicians before Wiles had shown that, if one could prove this curve was not modular, then this would prove Fermat’s Last Theorem!
That’s exactly what Wiles did. Despite being told that the proof would be impossible, he nonetheless persevered, drawing on the most intricate depths of group theory (the study of symmetries and their properties) that took over 129 pages to lay out.
It’s definitely not something that would have fit in Fermat’s margin! Nonetheless, we can now put down our pencils and stop searching for a cube that is the sum of two cubes, a fourth power that is the sum of two fourth powers, and so on.
And we must, for there are far bigger problems waiting for us. Tomorrow, we will meet what is probably the biggest one. In fact, it is so big, we will spend three days on it. It’s also worth $1,000,000—if you can prove it.
Stay tuned for our next stop: The Riemann Hypothesis.
Recommended book
Fermat’s Last Theorem by Simon Singh
Share with friends

