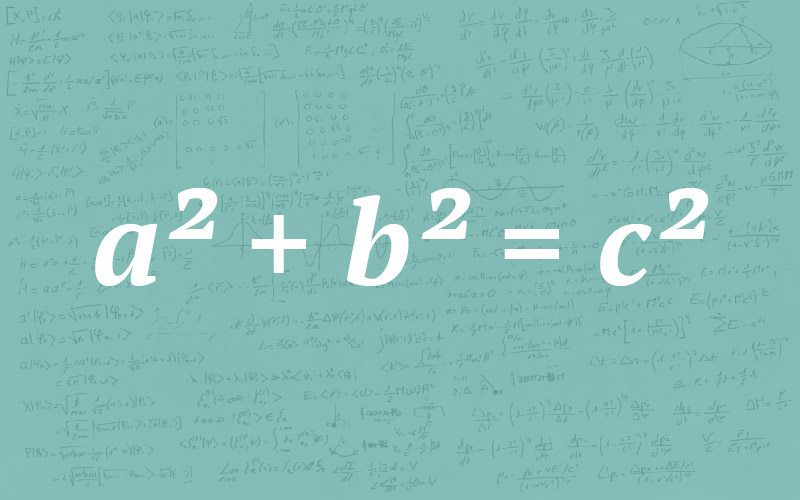The Pythagorean Theorem
Over 2000 years ago, the Greek mathematician Pythagoras developed a number of advancements in mathematical theory, especially in the field of geometry. The study of shapes and their relationships both in real space and mathematical abstractions, geometry is a mathematical field that follows strict guidelines and formulas to calculate information about each shape. Pythagoras formulated his Pythagorean Theorem to summarize an accurate pattern of calculation for triangles.
When two sides of a triangle are perpendicular to one another, they meet at a 90 degree angle. The third side of the triangle, called the hypotenuse, which is opposite the 90 degree angle and connects the other two sides of the triangle, will always be longer. This is called a “right triangle,” and the shorter sides are referred to as “a” and “b,” while the hypotenuse is labeled as “c.” Pythagoras figured out that a square formed from the hypotenuse would always be the size of squares formed from the other two sides of this triangle.
Therefore, the Pythagorean Theorem states that
a2 + b2 = c2
This basic formula holds true for any right triangle, with sides of any length, whether that triangle stands alone or is part of a larger geometric figure (such as a square, spiral or, fractal). The sum of the squares of the two shorter sides of a right triangle will always equal the squared number of the triangle’s longest side, its hypotenuse.
The development of this formula sparked new understandings of practical and theoretical geometry, affecting calculation that could be used in engineering as well as furthering computations in trigonometry and calculus. The Pythagorean Theorem has been applied to other shapes and serves as the basis for a number of other important mathematical formulas.
Expand your knowledge universe in just 5 minutes a day via bite-sized email courses.
Share with friends: