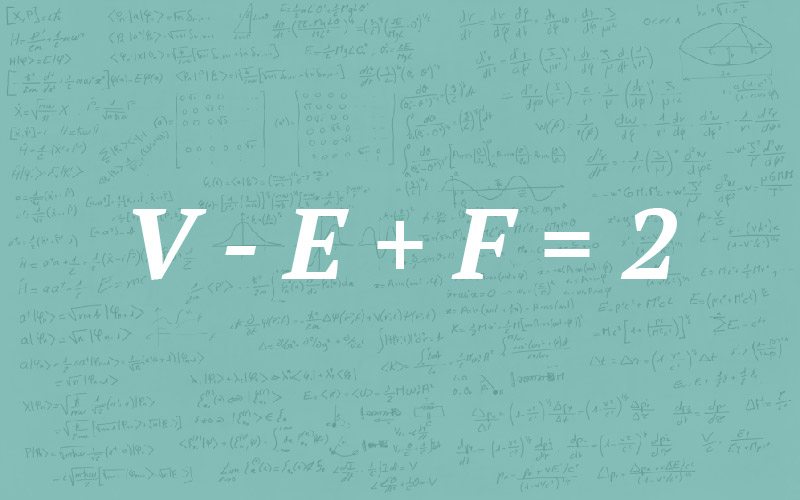Euler’s Formula for Polyhedra
Geometry is the mathematical field dealing with the properties of shapes. In three-dimensional space, it can be difficult to calculate information about shapes, such as their surface area or the angles of their edges. A three-dimensional shape such as a cube, cylinder, or sphere are each a common “polyhedron,” which means “many bases” or “many sides.” In particular a “convex polyhedron” is a three-dimensional shape that does not intersect itself and for which a line segment that connects any two points on the polyhedron can be contained within it. A soccer ball is one example of a common convex polyhedron.
For mathematicians, it was especially difficult to make calculations about irregularly-shaped polyhedra—until 18th century mathematician Leonhard Euler wrote a formula that works universally for all polyhedra.
Euler discovered that by using the number of vertices (V), edges (E), and faces (F) of a polyhedron, the calculation could more quickly and easily reveal the surface area of the shape. The classic Euler Characteristic is algebraically represented as:
χ = V – E + F
Building on this principle, Euler further discovered that by calculating the inverse of a convex polyhedron, the characteristic further demonstrates that:
V – E + F = 2
This formula is often known as Euler’s Polyhedron Formula, and it holds true for all convex simple polyhedra. A “simple” polyhedron is one which is solid and without any holes running through it. A hula hoop, car tire, or piece of tubing would all be considered “non-simple” polyhedra, and Euler’s Characteristic cannot be applied to calculate their surface area accurately.
The development of studying how polyhedra work in space sparked a new field of topography in geoscience, which studies many things about landscapes, including their mathematics. By using Euler’s Polyhedron Formula and applying it to natural shapes and formations, scientists can calculate information about the surface of those landscapes to use for structural engineering or preservation purposes.
Expand your knowledge universe in just 5 minutes a day via bite-sized email courses.
Share with friends: