The Golden Ratio

1.6180339887…

Episode #3 of the course “Most important numbers in the world”

In ancient mathematics, especially geometry, mathematicians ran across an important relationship between line segments and two-dimensional straight-lined shapes. In configuring quadrilaterals, pentagons, pentagrams, and other shapes, the proportion between certain lengths of the lines was not only most pleasing to the eye, but most structurally and mathematically sound. First described by Euclid in ancient Greece, the ratio now known as the “golden mean” is the sectioning of a line into an “extreme” mean of a specific proportion. Since the first descriptions and definitions of this predictable, repeatable relationship, mathematicians have been fascinated by its simplicity and its permeance through nature.

Also known as the “divine ratio” or the “golden number,” the golden ratio is often symbolized by the Greek letter “phi” (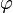 or ). It describes a relationship between a line and two of its segments, if the larger of the two segments is 1.6180 times the length of the smaller. When extrapolated into two-dimensional and three-dimensional shapes, the “golden ratio” becomes highly important for creating aesthetically and mathematically pleasing rectangles, columns, cylinders, and numerous polyhedra.

Artists and architects throughout history have been fascinated with the golden ratio, utilizing its proportions to create balance and perspective, movement, and enhanced geometrical emphasis in numerous paintings, sculptures, and buildings around the world. From classical Greek works of art and iconic architectural style came a number of revivals throughout history, each of which reappropriated the golden mean with renewed vigor. As mathematics grows to encompass more complex theories founded in the golden ratio, contemporary artists will continue to utilize this ancient constant relationship is new ways.

Share with friends